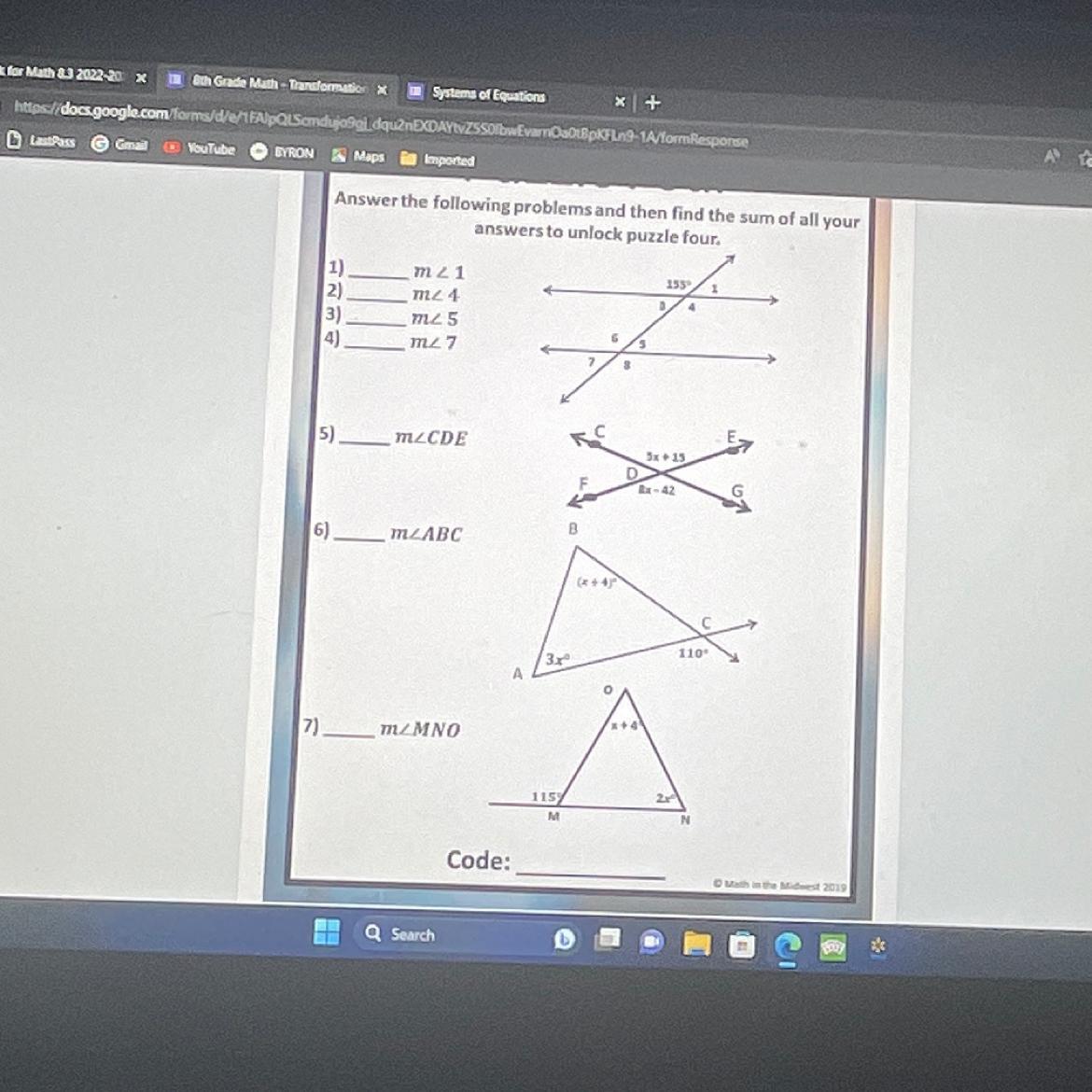
Answers
1) 25 degrees. 180-155= 25
2) 155 degrees. vertical Angles are the same
3) 25 degrees. same as 1
4) 25 degrees. vertical Angles 5 and 7
5) can't read it sry
I'm sorry I don't know the answers to the rest
Hope this helps. if u need any other help understanding then just message me through this app
Related Questions
(a) Prove the product rule for complex functions. More specifically, if f(z) and g(z) are analytic prove that h(z) = f(z)g(z) is also analytic, and that h'(z) = f'(z)g(z) + f(z)g′(z). (b) Let Sn be the statement d = nzn-1 for n N = = {1, 2, 3, ...}. da zn If it is established that S₁ is true. With the help of (a), show that if Sn is true, then Sn+1 is true. Why does this establish that Sn is true for all n € N?
Answers
(a) To prove the product rule for complex functions, we show that if f(z) and g(z) are analytic, then their product h(z) = f(z)g(z) is also analytic, and h'(z) = f'(z)g(z) + f(z)g'(z).
(b) Using the result from part (a), we can show that if Sn is true, then Sn+1 is also true. This establishes that Sn is true for all n € N.
(a) To prove the product rule for complex functions, we consider two analytic functions f(z) and g(z). By definition, an analytic function is differentiable in a region. We want to show that their product h(z) = f(z)g(z) is also differentiable in that region. Using the limit definition of the derivative, we expand h'(z) as a difference quotient and apply the limit to show that it exists. By manipulating the expression, we obtain h'(z) = f'(z)g(z) + f(z)g'(z), which proves the product rule for complex functions.
(b) Given that S₁ is true, which states d = z⁰ for n = 1, we use the product rule from part (a) to show that if Sn is true (d = nzn-1), then Sn+1 is also true. By applying the product rule to Sn with f(z) = z and g(z) = zn-1, we find that Sn+1 is true, which implies that d = (n+1)zn. Since we have shown that if Sn is true, then Sn+1 is also true, and S₁ is true, it follows that Sn is true for all n € N by induction.
In conclusion, by proving the product rule for complex functions in part (a) and using it to show the truth of Sn+1 given Sn in part (b), we establish that Sn is true for all n € N.
To learn more about product rule click here: brainly.com/question/29198114
#SPJ11
The relationship between the velocity, U, of a construction vehicle (in km/h) and the distance, d (in metre), required to bring it to a complete stop is known to be of the form d = au? + bu + C, where a, b, and c are constants. Use the following data to determine the values of a, b, and c when: a) U = 20 and d = 40 b) u = 55, and d = 206.25 c) U = 65 and d = 276.25 [Note: Use an appropriate standard engineering software such as MATLAB, CAS calculator, programmable calculator, Excel software)
Answers
To determine the values of the constants a, b, and c in the relationship between velocity U and stopping distance d, we can use the given data points and solve a system of equations.
Let's substitute the given values into the equation d = au^2 + bu + c:
For data point a) U = 20 and d = 40:
[tex]\[40 = a \cdot 20^2 + b \cdot 20 + c\][/tex]
For data point b) U = 55 and d = 206.25:
[tex]\[206.25 = a \cdot 55^2 + b \cdot 55 + c\][/tex]
For data point c) U = 65 and d = 276.25:
[tex]\begin{equation}276.25 = a(65)^2 + b(65) + c\end{equation}[/tex]
We now have a system of three equations in three variables (a, b, c). By solving this system, we can find the values of a, b, and c that satisfy all three equations simultaneously.
You can use appropriate software such as MATLAB, CAS calculator, programmable calculator, or Excel to solve the system of equations and find the values of a, b, and c. These software tools have built-in functions or methods for solving systems of equations numerically.
Once you have the solutions for a, b, and c, you can substitute them back into the original equation to obtain the complete relationship between velocity U and stopping distance d.
To know more about velocity visit:
https://brainly.com/question/14534415
#SPJ11
A fireman’s ladder leaning against a house makes an angle of 62 with the ground. If the ladder is 3 feet from the base of the house, how long is the ladder?
Answers
In the given scenario ladder is 6.52 feet long.
Given that,
The angle between ground and ladder = 62 degree
The distance of ladder from ground and ladder = 3 feet
We have to find the length of ladder.
Since we know that,
The trigonometric ratio
cosθ = adjacent/ Hypotenuse
Here we have,
Adjacent = 3 feet
Hypotenuse = length of ladder
Thus to find the length of ladder we have to find the value of hypotenuse.
Therefore,
⇒ cos62 = 3/ Hypotenuse
⇒ 0.46 = 3/ Hypotenuse
⇒ Hypotenuse = 3/0.46
= 6.52
Thus,
length of ladder = 6.52 feet.
Learn more about the triangle visit;
brainly.com/question/1058720
#SPJ1
Use a graphing utility to graph the function and find the absolute extrema of the function on the given interval. (Round your answers to three decimal places. If an answer does not exist, enter DNE.) f(x) -x4 - 2x3 + x +1, I-1, 3]
Answers
The absolute extrema of the function on the given interval using the graphing utility, are as follows:
Absolute maximum value = 3
Absolute minimum value = -5.255
A graphing utility, also known as a graphing calculator or graphing software, is a tool that allows users to create visual representations of mathematical functions, equations, and data. It enables users to plot graphs and analyze various mathematical concepts and relationships visually.
To use a graphing utility to graph the function and find the absolute extrema of the function on the given interval, follow these steps:
1.Graph the function on the given interval using a graphing utility. We get this graph:
2.Observe the endpoints of the interval. At x = -1, f(x) = 3 and at x = 3, f(x) = -23.
3.Find critical points of the function, which are points where the derivative is zero or does not exist.
Differentiate the function: f'(x) = -4x³ - 6x² + 1.
We set f'(x) = 0 and solve for x.
Then we factor the equation. -4x³ - 6x² + 1 = 0 → x = -0.962, -0.308, 1.256.
These are the critical points.
4.Find the value of the function at each of the critical points.
We use the first derivative test or the second derivative test to determine whether each critical point is a maximum, a minimum, or an inflection point.
When x = -0.962, f(x) = 1.373.When x = -0.308, f(x) = 1.079.
When x = 1.256, f(x) = -5.255.5.
Compare the values at the endpoints and the critical points to find the absolute maximum and minimum of the function on the interval [-1, 3].
The absolute maximum value is 3, which occurs at x = -1.
The absolute minimum value is -5.255, which occurs at x = 1.256.
Therefore, the absolute extrema of the function on the given interval are as follows:
Absolute maximum value = 3
Absolute minimum value = -5.255
To know more about graphing utility, visit:
https://brainly.com/question/28323287
#SPJ11
1.10
Exercises 1.
1. Show that if q = mr/r3, where m is a constant, the equation of continuity for an incompressible fluid is satisfied at all points except the origin.
2. State the restriction that must be placed on the constants a, b, c, d in order that the vector field (az + by)+(cz+dy)} can be expressed as the gradient of a scalar.
Answers
The necessary restriction on the constants a, b, c, and d for the vector field (az + by) + (cz + dy) to be expressible as the gradient of a scalar is a = b = c = 0.
1. To show that the equation of continuity for an incompressible fluid is satisfied at all points except the origin for the vector field [tex]q = (mr/r^3)[/tex], where m is a constant, we need to consider the divergence of the vector field.
The continuity equation for an incompressible fluid states that the divergence of the velocity field is zero. Mathematically, it can be written as:
∇ · v = 0
Here, v represents the velocity vector field. In this case, we are given [tex]q = (mr/r^3)[/tex], which is related to the velocity field v.
Let's find the divergence of q using the expression:
∇ · q = ∇ · [tex](mr/r^3)[/tex]
Using the product rule of divergence, we have:
∇ · q = [tex](1/r^3)[/tex]∇ · (mr) + m∇ · [tex](1/r^3)[/tex]
The first term on the right side can be simplified as:
∇ · (mr) = (∇m) · r + m∇ · r
Since m is a constant, its gradient is zero (∇m = 0). Additionally, the divergence of the position vector (∇ · r) is equal to 3/r, where r represents the magnitude of the position vector.
∇ · (mr) = 0 + m(3/r) = 3m/r
Now let's simplify the second term:
∇ · (1/r^3) = ∇ · (r^{-3})
Using the chain rule for divergence, we get:
∇ · [tex](1/r^3)[/tex] = [tex](-3r^{-4})[/tex](∇ · r) = [tex](-3/r^4)(3/r)[/tex] = [tex]-9/r^5[/tex]
Substituting these results back into the expression for ∇ · q, we have:
∇ · q = [tex](1/r^3)(3m/r)[/tex] + [tex]m(-9/r^5)[/tex]
Simplifying further, we get:
∇ · q = [tex]3m/r^4 - 9m/r^6[/tex]
Now let's consider the points where this equation is satisfied. At any point where ∇ · q = 0, the equation of continuity is satisfied.
Setting ∇ · q = 0, we have:
[tex]3m/r^4 - 9m/r^6 = 0[/tex]
[tex]1/r^4 - 3/r^6 = 0[/tex]
[tex]r^2 - 3 = 0[/tex]
This equation has two roots: r = √3 and r = -√3. However, since we are considering physical positions in space, the radial distance r cannot be negative. Therefore, the only valid solution is r = √3.
Hence, the equation of continuity is satisfied at all points except the origin (r = 0) for the vector field q = ([tex]mr/r^3[/tex]), where m is a constant.
2. In order for the vector field F = (az + by) + (cz + dy) to be expressible as the gradient of a scalar function, certain restrictions must be placed on the constants a, b, c, and d. The necessary condition is that the vector field F must be conservative.
For a vector field to be conservative, its curl (denoted as ∇ × F) must be zero. Mathematically, this condition can be expressed as:
∇ × F = 0
Let's calculate the curl of F:
∇ × F = ∇ × [(az + by) + (cz + dy)]
Using the properties of curl, we can split this into two separate curls:
∇ × F = ∇ × (az + by) + ∇ × (cz + dy)
For the first term, ∇ × (az + by), we can use the fact that the curl of the gradient of any scalar function is zero:
∇ × ∇φ = 0, where φ is a scalar function
Therefore, the first term vanishes:
∇ × (az + by) = 0
For the second term, ∇ × (cz + dy), we calculate the curl using the components:
∇ × (cz + dy) = (∂(dy)/∂x - ∂(cz)/∂y) i + (∂(cz)/∂x - ∂(dy)/∂z) j + (∂(dy)/∂z - ∂(cz)/∂y) k
Comparing the components of the curl with the components of the vector field F, we get:
∂(dy)/∂x - ∂(cz)/∂y = a
∂(cz)/∂x - ∂(dy)/∂z = b
∂(dy)/∂z - ∂(cz)/∂y = c
From these equations, we can see that for F to be conservative (curl = 0), the following conditions must be satisfied:
a = 0
b = 0
c = 0
Hence, the restrictions on the constants a, b, c, and d are a = b = c = 0, in order for the vector field (az + by) + (cz + dy) to be expressible as the gradient of a scalar function.
To know more about vector field, refer here:
https://brainly.com/question/32574755
#SPJ4
1. The multiplier used for constructing a 97% confidence interval for population proportion p using a sample of size 28 is _______
(you need to find p)
2. To construct a 95% confidence interval for a population mean with a margin of error of 0.3 from a Normal population that has standard deviation =4.7σ=4.7, one would need a sample size of ________
Answers
The multiplier used for constructing a 97% confidence interval for population proportion p using a sample of size 28 is 2.1701.2.
:Given,Sample size n = 28Level of confidence = 97%To find: Multiplier used for constructing a 97% confidence interval for population proportion pFormula used to find the multiplier is given as, Multiplier = Zα/2Where Zα/2 is the standard normal random variable at α/2 level of significance
Summary:Sample size needed to construct a 95% confidence interval for a population mean with a margin of error of 0.3 from a Normal population that has standard deviation =4.7σ=4.7 is 34.31.
Learn more about standard deviation click here:
https://brainly.com/question/475676
#SPJ11
the pdf has ab exponential random variable x is: what is the expected value of x?
Answers
The expected value of an exponential random variable x is equal to the inverse of the parameter λ.
The exponential distribution is a probability distribution that describes the time between events in a Poisson process, where events occur continuously and independently at a constant average rate λ.
The probability density function (pdf) of an exponential random variable x is given by:
f(x) = λe^(-λx)
To calculate the expected value of x, denoted as E(x) or μ, we integrate x times the pdf over the entire range of x:
E(x) = ∫[0 to ∞] x * λe^(-λx) dx
Integrating the expression, we obtain:
E(x) = -x * e^(-λx) - (1/λ)e^(-λx) | [0 to ∞]
E(x) = [0 - (-0) - (1/λ)e^(-λ∞)] - [0 - (-0) - (1/λ)e^(-λ0)]
Since e^(-λ∞) approaches 0 as x goes to infinity and e^(-λ0) equals 1, the expression simplifies to:
E(x) = (1/λ)
Therefore, the expected value of an exponential random variable x is equal to the inverse of the parameter λ.
To know more about exponential distribution, refer here:
https://brainly.com/question/30669822#
#SPJ11
Find an equation of the tangent line to the graph of the function f(x) = 2-3 at the point (-1,2). Present the equation of the tangent line in the slope-intercept form y = math.
Answers
We have the function: f(x) = 2 - 3xWe are required to find an equation of the tangent line to the graph of the function at the point (-1,2).To find the tangent line, we need to find the slope and the point (-1, 2) lies on the tangent line.
Now we have a slope and a point (-1,2). We can use the point-slope form of a line to find the equation of the tangent line.y - y₁ = m(x - x₁)where m is the slope and (x₁, y₁) is the point on the line. Plugging in the values, we have:y - 2 = -3(x + 1)Simplifying, we get:y = -3x - 1
Thus, the required equation of the tangent line in slope-intercept form is:y = -3x - 1
To know more about equation visit:
https://brainly.com/question/29657983
#SPJ11
The population of fish in a farm-stocked lake after t years could be modeled by the equation.
P(t( = 1000/1+9e-0.6t (a) Sketch a graph of this equation. (b) What is the initial population of fish?
Answers
(a) The graph of the given equation[tex]P(t) = 1000/1 + 9e^(-0.6t)[/tex] can be drawn using the following steps. Step 1: Plot the point (0, 100) which is the initial population of fish. Step 2: Choose some values for t and find out the corresponding values of P(t). Step 3: Plot the ordered pairs obtained from the values of t and P(t).Step 4: Connect the plotted points to obtain the graph of the equation.
(b) We are given the population equation for a farm-stocked lake as P(t) = 1000/1 + 9e^(-0.6t). In order to find the initial population of fish, we substitute t = 0 in the given equation. [tex]P(0) = 1000/1 + 9e^(0)[/tex]
= 1000/10
= 100.
The initial population of fish is 100.
To know more about ordered pairs visit:
https://brainly.com/question/30805001
#SPJ11
"
Let p = 31 (a) How many primitive roots are there mod 31? (b) Is 2 a primitive root? Explain. (c) Is 3 a primitive root? Explain. (d) Using the order formula, find all the elements of order 6
Answers
The elements of order 6 are (15^5, 15^17, 16^2, 16^8, 18^5, 18^17) where p = 31.
(a) How many primitive roots are there mod 31?
To solve the given problem, we know that a is a primitive root of p if and only if a is a generator of the group of units modulo p.
Then by the formula of Euler's totient function,
φ(31) = 30 since 31 is prime.
Therefore the group of units modulo 31 has φ(30) = 8 primitive roots.
b) Is 2 a primitive root?
The order of 2 is 15, not 30. 2^(15) ≡ −1 mod 31, which means that 2 is not a primitive root modulo 31.
c) Is 3 a primitive root?
The order of 3 is 5 since 3^(5) ≡ −1 mod 31.
Therefore, 3 is a primitive root of 31.
d) Using the order formula, find all the elements of order 6?
Let us consider an element "a" and let "k" be the smallest positive integer such that a^(k) = 1 mod p.
Then "k" is called the order of a mod p.
Using the order formula, the elements of order 6 are:
For k = 6: (15^5, 15^17, 16^2, 16^8, 18^5, 18^17).
Therefore, all the elements of order 6 are (15^5, 15^17, 16^2, 16^8, 18^5, 18^17) where p = 31.
To learn more about function, visit the link below
https://brainly.com/question/30721594
#SPJ11
Consider the following 5 statements. 2 of the statements are false in general. Determine which 2 statements are false by testing out each statement on an appropriate matrix (like we did with the properties of determinants in Section 3.3 of the tutorial file) Note: You should not use a magic or pascal matrix for (i) or (ii) below because they have special properties not shared by other matrices. Try using rand instead (i) If A is nx n, then A and A1 have the same eigenvalues (ii) If A is n × n, then A and A-1 have the same eigenvectors (iii) If A is n × n then det(Ak) = [det(A)]k (iv) If I is the n×n identity matrix, and J 1s an n x n matrix consisting entirely of ones, then the matrixis nvertible and (1-+J. (v) If I is the n×n identity matrix, and J 1s an n×n matrix consisting entirely of ones, then the matrix A = 1-..T 1S ide I is idempotent (i.e,A2-/A) Don't forget that you are selecting which statements are false (you are not selecting which statements are true) (A) (i) and (v) (B) (iii) and (v) (C) (ii) and (v) (D) (iii) and (iv) (E) (ii) and (iv) (F) (i) and (iv) (G) (iv) and (v) (H) (i) and (ii)
Answers
The two false statements among the five given statements are (i) and (iii).
The proof for each statement is given below.
(i) If A is nx n, then A and A1 have the same eigenvalues: This statement is false in general, as a matrix and its inverse have the same eigenvalues, but A and A1 are not inverses of each other.
We can test this statement using the rand(n) command in MATLAB.
Consider the matrix A = rand(3)
Then, we can calculate the eigenvalues of A using eig(A)
This gives the outputans
=3.0677+0.0000i-0.0833+0.9025i-0.0833-0.9025i
Next, we can calculate the eigenvalues of A1, which is simply the inverse of A.
For this, we can use the inv() command in MATLAB. eig(inv(A))
This gives the outputans
=0.3255+0.0000i0.0045+0.2107i0.0045-0.2107i
Clearly, the eigenvalues of A and A1 are not the same.
(ii) If A is n × n, then A and A-1 have the same eigenvectors: This statement is true in general, as a matrix and its inverse have the same eigenvectors.
We can test this statement using the rand(n) command in MATLAB.
Consider the matrix A = rand(3)
Then, we can calculate the eigenvectors of A using eig(A)
This gives the outputans
=3.0677+0.0000i-0.0833+0.9025i-0.0833-0.9025i
The first column of V is an eigenvector corresponding to the first eigenvalue, and so on.
Next, we can calculate the eigenvectors of A1, which is simply the inverse of A. For this, we can use the inv() command in MATLAB. eig(inv(A))
This gives the outputans
=0.3255+0.0000i0.0045+0.2107i0.0045-0.2107i
The first column of V is an eigenvector corresponding to the first eigenvalue, and so on.
(iii) If A is n × n, then det(Ak) = [det(A)]k: This statement is false in general, as the determinant of a matrix raised to a power is not equal to the determinant of the matrix raised to the same power.
We can test this statement using the rand(n) command in MATLAB. Consider the matrix A = rand(3)
Then, we can calculate the determinant of A using det(A)
This gives the outputans =0.0876
Next, we can calculate the determinant of Ak, where k = 2, for example.
For this, we can use the det() command in MATLAB. det(A^2)
This gives the outputans =0.0129
Clearly, det(Ak) ≠ [det(A)]k.
Therefore, the false statements are (i) and (iii), which means that the correct answer is option (A) (i) and (v).
Know more about the eigenvalues,
https://brainly.com/question/2289152
#SPJ11
As F gets larger than, , we can start to detect differences between treatment groups over the noise. Type your answer.... 17 2 points Which of the following values of the chi-square test statistic would be most likely to suggest that the null hypothesis was really true?
Answers
None of the following values of the chi-square test statistic would be most likely to suggest that the null hypothesis was really true. As F gets larger than 1, we can start to detect differences between treatment groups over the noise.
ANOVA (Analysis of Variance) is a method of testing for a difference between three or more population means that is commonly employed in various statistical applications.
It is the F-statistic that provides the level of significance of the test in ANOVA. As F gets larger than 1, we can start to detect differences between treatment groups over the noise.
The chi-square test statistic is used to test whether the observed data matches a distribution's expected data, or to determine whether there is a relationship between two variables.
To conclude, none of the following values of the chi-square test statistic would be most likely to suggest that the null hypothesis was really true.
To know more about chi-square test , refer
https://brainly.com/question/4543358
#SPJ11
Prove that for f continues it is worth [ƒ [ dv f dV = f(xo) dV A A for some xo E A.
Answers
To prove that for a continuous function f, it is worth [ƒ [ dv f dV = f(xo) dV A A for some xo E A, we can use the mean value theorem for integrals.
Let A be a bounded set in R^n, and let f be a continuous function on A. Then, there exists a point xo in A such that
∫A f(x) dV = f(xo) * V(A)
where V(A) is the volume (or area) of A.
To see why this is true, consider the function g(t) = ∫A f(x) dt, where A is fixed and x is a variable in A. By the fundamental theorem of calculus, g'(t) = f(x(t)) * dx/dt, where x(t) is a path in A. Since f is continuous, it is integrable, and so g is differentiable by the Leibniz rule for differentiation under the integral sign. Thus, by the mean value theorem for integrals, there exists a value t0 in [0,1] such that
∫A f(x) dV = g(1) - g(0) = g'(t0) = f(x0) * V(A)
where x0 = x(t0) is a point in A.
Therefore, for any continuous function f on a bounded set A, we can always find a point xo in A such that [ƒ [ dv f dV = f(xo) dV A A.
To know more about continuous function visit:
https://brainly.com/question/28228313
#SPJ11
Suppose that p(x) = c/3*, x = 1,2,..., is the probability function for a random variable X. 35. Determine c. (a) 2 (b) 2.25 (c) 1.5 (d) 1.8 36. Find P(2 ≤X<5) 26 (a) 81 13 (b) 13 (c) 54 13 (d) 45 37. Which of the following is a false property of a standard normal distribution? I: the mean is zero (0) and the standard deviation is 1. II: the distribution is symmetric about the mean. III: the mean, mode and median are the same. IV: P(-1 ≤Z≤ 1)=0.68. (a) I only (b) IV only (c) All the above (d) None of the above.
Answers
The correct option is `(c) All the above`.None of the properties is false.
We are given that the probability function for a random variable X is given by,[tex]`p(x) = c/3*, x = 1,2,...,`[/tex]
We are to determine the value of c. Given probability function is [tex]`p(x) = c/3*`.[/tex]
The sum of probabilities of all the events is 1.
So, we can use this concept to find the value of c.[tex]`P(X = 1) + P(X = 2) + P(X = 3) + ... = 1`[/tex]
We know that the probability function is given as,[tex]`p(x) = c/3*[/tex]
`When [tex]`x = 1`, `p(x = 1) = c/3`[/tex]
When `[tex]x = 2`, `p(x = 2) = c/3*2[/tex]
`When[tex]`x = 3`, `p(x = 3) = c/3*3[/tex]
When `x = n`, `p(x = n) = c/3*n`
Therefore,[tex]`P(X = 1) + P(X = 2) + P(X = 3) + ... = c/3 + c/3*2 + c/3*3 + ... = 1[/tex]
`Let's simplify the equation.[tex]`c/3 + c/3*2 + c/3*3 + ... = 1``c/3(1 + 1/2 + 1/3 + ...) = 1``c/3ln(e) = 1``c = 3/ln(e)`[/tex]
Hence, the value of c is `3/ln(e)`.We are given that `p(x) = c/3*` and we need to find [tex]`P(2 ≤X < 5)`.`P(2 ≤X < 5) = P(X = 2) + P(X = 3) + P(X = 4)`[/tex]
From part (a), we know that `c = 3/ln(e)`.
Therefore,[tex]`p(x) = (3/ln(e))/(3*x)``P(X = 2) \\= (3/ln(e))/(3*2) = 0.5/ln(e)``P(X = 3) \\=(3/ln(e))/(3*3) = 0.5/ln(e)``P(X = 4) \\= (3/ln(e))/(3*4) = 0.5/ln(e)`[/tex]
Hence,[tex]`P(2 ≤X < 5) = P(X = 2) + P(X = 3) + P(X = 4) = 0.5/ln(e) + 0.5/ln(e) + 0.5/ln(e) \\= 1.5/ln(e)`[/tex]
Hence, the required probability is `1.5/ln(e)`.
We need to determine the false property of a standard normal distribution.
We know that a standard normal distribution has mean `μ = 0` and standard deviation `σ = 1`. T
he distribution is symmetric about the mean. The mean, mode, and median are the same.
The probability of getting a value between `-1` and `1` is `0.68`.
Therefore, the correct option is `(c) All the above`.None of the properties is false.
Know more about probability here:
https://brainly.com/question/25839839
#SPJ11
Review and discuss the difference between statistical
significance and practical significance.
Answers
Statistical significance and practical significance are two important concepts in statistical analysis and research.
How are statistical and practical significance different ?Statistical significance refers to the probability that an observed effect or difference in a dataset is not attributable to random chance. It is determined through statistical tests, such as hypothesis testing, where researchers juxtapose the observed data to an anticipated distribution under the null hypothesis.
Conversely, practical significance centers on the practical or real-world importance and meaningfulness of an observed effect. It transcends statistical significance and assesses whether the observed effect holds any practical or substantive relevance.
Find out more on practical significance at https://brainly.com/question/30311816
#SPJ4
Which of the following is the Maclaurin series representation of the function f(x) = (1+x)3?
a) Σ n=1 n (n + 1) 2 x", -1
b) Σ B n=1 (n+1)(n+2) 2 x+1, -1
c) Σ (-1)"¹n (n+1) x"+¹¸ −1
d) Σ (-1)-(n+1)(n+2) x", −1
Answers
A three-dimensional vector, also known as a 3D vector, is a mathematical object that represents a quantity or direction in three-dimensional space.
To solve initial-value problems using Laplace transforms, you typically need well-defined equations and initial conditions. Please provide the complete and properly formatted equations and initial conditions so that I can assist you further.
For example, a 3D vector v = (2, -3, 1) represents a vector that has a magnitude of 2 units in the positive x-direction, -3 units in the negative y-direction, and 1 unit in the positive z-direction.
3D vectors can be used to represent various physical quantities such as position, velocity, force, and acceleration in three-dimensional space. They can also be added, subtracted, scaled, linear algebra, and computer graphics.
To know more about the equation:- https://brainly.com/question/29657983
#SPJ11
Find a particular solution to the differential equation using the Method of Undetermined Coefficients. d²y / dx² - 3 dy/dx +4y= x e^x
Answers
The general solution of the given differential equation is given by: [tex]`y(x) = y_c(x) + y_p(x)``y(x) \\= c₁ e^(3x/2) cos(√7x/2) + c₂ e^(3x/2) sin(√7x/2) + xe^x`[/tex]
Given differential equation:[tex]`d²y / dx² - 3 dy/dx +4y= x e^x`.[/tex]
Particular solution to the differential equation using the Method of Undetermined CoefficientsTo find the particular solution to the differential equation using the method of undetermined coefficients, we need to follow the steps below:
Step 1: Find the complementary function of the differential equation.
We solve the characteristic equation of the given differential equation to obtain the complementary function of the differential equation.
Characteristic equation of the given differential equation is[tex]: `m² - 3m + 4 = 0`[/tex]
Solving the above equation, we get,[tex]`m = (3 ± √(-7))/2``m = (3 ± i√7)/2`[/tex]
Therefore, the complementary function of the given differential equation is given by: [tex]`y_c(x) = c₁ e^(3x/2) cos(√7x/2) + c₂ e^(3x/2) sin(√7x/2)`[/tex]
Step 2: Find the particular solution of the differential equation by assuming the particular solution has the same form as the non-homogeneous part of the differential equation.
Assuming[tex]`y_p = (A + Bx) e^x`.[/tex]
Hence,[tex]`dy_p/dx = Ae^x + (A + Bx) e^x` and `d²y_p / dx² = 2Ae^x + (A + 2B) e^x`[/tex]
Substituting these values in the differential equation, we get:`
[tex]d²y_p / dx² - 3 dy_p/dx + 4y_p = x e^x`\\⇒ `2Ae^x + (A + 2B) e^x - 3Ae^x - 3(A + Bx) e^x + 4(A + Bx) e^x \\= x e^x`⇒ `(A + Bx) e^x \\= x e^x`[/tex]
Comparing the coefficients, we get,`A = 0` and `B = 1`
Therefore, `[tex]y_p = xe^x`[/tex].
Hence, the particular solution of the given differential equation is given by[tex]`y_p(x) = xe^x`.[/tex]
Therefore, the general solution of the given differential equation is given by:[tex]`y(x) = y_c(x) + y_p(x)``y(x) \\= c₁ e^(3x/2) cos(√7x/2) + c₂ e^(3x/2) sin(√7x/2) + xe^x`[/tex]
Know more about differential equation here:
https://brainly.com/question/1164377
#SPJ11
Consider the map 0:P2 P2 given by → (p(x)) = p(x) - 2(x + 3)p'(x) - xp"() ('(x) is the derivative of p(x) etc). Let S = {1, x, x2} be the standard basis of P2, and let B = {P1 = 1+x+x2, P2 = 2 - 2x + x2, P3 = x - x?}. Show: 1) B is a basis of P, and give the transition matrix P = Ps<--B 2) Show o is linear and give the matrix A = [ø]s of the linear map in the basis S. 3) Find the matrix A' = [0]B of the linear map o in the basis B.
Answers
Here, [0]B = [-2 -2 0] with respect to the basis B.
1) To show that B is a basis of P2, we can show that the vectors in B are linearly independent and span P2.
Linear independence:
To show linear independence, let α1P1 + α2P2 + α3P3 = 0 for some α1, α2, α3 ∈ R.
Then we have
(α1 + 2α2 + α3) + (α1 - 2α2 + α3)x + (α1 + α2 - α3)x2 = 0
for all x ∈ R. In particular, we can evaluate this at x = 0, 1, and -1.
At x = 0, we get α1 + 2α2 + α3 = 0.
At x = 1, we get α1 = 0. Finally, at x = -1, we get -α1 + α2 - α3 = 0.
Putting these together, we get α1 = α2 = α3 = 0.
Therefore, B is linearly independent.
Span:
To show that B spans P2, we can show that any polynomial p(x) ∈ P2 can be written as a linear combination of the vectors in B.
Let p(x) = a + bx + cx2. Then we have
a + bx + cx2 = (a + b + c)P1 + (2 - 2b + c)P2 + (b - c)P3
Therefore, B is a basis of P2.
We can find the transition matrix P = Ps<-B as the matrix whose columns are the coordinate vectors of P1, P2, and P3 with respect to the basis B.
We have
P = [1 2 0; 1 -2 1; 1 1 -1]2)
To show that o is linear, we need to show that for any polynomials p(x), q(x) ∈ P2 and any scalars a, b ∈ R, we have
o(ap(x) + bq(x)) = aop(x) + boq(x).
Let's do this now:
First, let's compute op(x) for each p(x) ∈ S. We have
o(1) = 1 - 2(3) = -5o(x) = x - 2 = -2 + xo(x2) = x2 - 2(2x) - x = -x2 - 2x
Therefore, [ø]s = [-5 -2 -1]
Finally, to find the matrix A' = [0]
B of the linear map o in the basis B, we need to find the coordinates of
o(P1), o(P2), and o(P3) with respect to the basis B.
We have
o(P1) = o(1 + x + x2)
= -5 - 2(2) - 1(-1)
= -2o(P2) = o(2 - 2x + x2)
= -5 - 2(-2) - 1(1)
= -2o(P3)
= o(x - x2)
= -(-1)x2 - 2x = x2 + 2x
Therefore, [0]B = [-2 -2 0]
To know more about basis visit:
https://brainly.com/question/30451428
#SPJ11
Assuming that a 9:3:1 three-class weighting sys- tem is used, determine the central line and control limits when Uoc = 0.08, loma = 0.5, Uomi = 3.0, and n = 40. Also calculate the demerits per unit for May 25 when critical nonconformities are 2, major noncon- formities are 26, and minor nonconformities are 160 for the 40 units inspected on that day. Is the May 25 subgroup in control or out of control?
Answers
To determine the central line and control limits for a 9:3:1 three-class weighting system, the following values are needed: Uoc (Upper Operating Characteristic), loma (Lower Operating Minor), Uomi (Upper Operating Major), and n (sample size).
The central line in a 9:3:1 three-class weighting system is calculated as follows:
Central Line = (9 * Critical Nonconformities + 3 * Major Nonconformities + 1 * Minor Nonconformities) / Total Number of Units Inspected
The upper control limit (UCL) and lower control limit (LCL) can be determined using the following formulas:
UCL = Central Line + Uoc * √(Central Line / n)
LCL = Central Line - loma * √(Central Line / n)
To calculate the demerits per unit, the following formula is used:
Demerits per Unit = (9 * Critical Nonconformities + 3 * Major Nonconformities + 1 * Minor Nonconformities) / Total Number of Units Inspected To assess whether the May 25 subgroup is in control, we compare the demerits per unit for that day with the control limits. If the demerits per unit fall within the control limits, the subgroup is considered to be in control. Otherwise, it is considered out of control.
Learn more about demerits here: brainly.com/question/32238590
#SPJ11
find a power series representation for the function and determine the interval of convergence. (give your power series representation centered at x = 0.) f(x)=1/(6 x)
Answers
The power series representation of f(x) is f(x) = (1/6) * (1 - x/6 + x²/36 - x³/216 + ...) and centered at x = 0. Also, the interval of convergence for the power series representation.
Understanding Power SeriesThe function f(x) = 1/(6x) can be represented as a power series using the geometric series formula. Recall that the geometric series formula is:
1 / (1 - r) = 1 + r + r² + r³ + ...
In this case, we can rewrite f(x) as:
f(x) = 1/(6x) = (1/6) * (1/x) = (1/6) * (1/(1 - (-x/6)))
Now, we can identify that the function is in the form of a geometric series with a common ratio of -x/6. Therefore, we can use the geometric series formula to write f(x) as a power series:
f(x) = (1/6) * (1/(1 - (-x/6)))
= (1/6) * (1 + (-x/6) + (-x/6)² + (-x/6)³ + ...)
Simplifying the expression:
f(x) = (1/6) * (1 - x/6 + x²/36 - x³/216 + ...)
This is the power series representation of f(x) centered at x = 0.
To determine the interval of convergence, we need to find the values of x for which the power series converges. In this case, the power series is a geometric series, and we know that a geometric series converges when the absolute value of the common ratio is less than 1.
In our power series, the common ratio is -x/6. So, for convergence, we have:
|-x/6| < 1
Taking the absolute value of both sides:
|x/6| < 1
-1 < x/6 < 1
-6 < x < 6
Therefore, the interval of convergence for the power series representation of f(x) is -6 < x < 6.
Learn more about power series here:
https://brainly.com/question/28158010
#SPJ1
Find the limit if it exists. lim (2x+1) X-14 Select the correct choice below and, if necessary, fill in the answer box to complete your choice. A. lim (2x+1)= (Simplify your answer.) x-4 B. The limit does not exist.
Answers
The limit of (2x+1)/(x-14) as x approaches 14 is A. lim (2x+1) = 29. To find the limit, we can directly substitute the value 14 into the expression (2x+1)/(x-14).
However, this leads to an indeterminate form of 0/0. To resolve this, we can factor the numerator as 2x+1 = 2(x-14) + 29.
Now, we can rewrite the expression as (2(x-14) + 29)/(x-14). Notice that the term (x-14) in the numerator and denominator cancels out, resulting in 2 + 29/(x-14).
As x approaches 14, the value of (x-14) approaches 0. Therefore, the limit of (2(x-14) + 29)/(x-14) is equal to 2 + 29/0, which is undefined.
Hence, the correct choice is B. The limit does not exist, as the expression approaches an undefined value as x approaches 14.
Learn more about indeterminate form here: brainly.com/question/30640456
#SPJ11
Normal Distribution
The time needed to complete a quiz in a particular college course is normally distributed with a mean of 160 minutes and a standard deviation of 25 minutes. What is the probability of completing the quiz in 120 minutes or less? and What is the probability that a student will complete it in more than 120 minutes but less than 150 minutes?
Answers
The probability of completing the quiz in 120 minutes or less is 0.2119 and in more than 120 minutes but less than 150 minutes is 0.1056.
What are the probabilities for quiz completion?The completion time of the quiz in this college course follows a normal distribution with a mean of 160 minutes and a standard deviation of 25 minutes. To calculate the probability of completing the quiz in 120 minutes or less, we need to find the area under the normal curve to the left of 120 minutes. By standardizing the value using the z-score formula (z = (x - mean) / standard deviation), we find that the z-score for 120 minutes is -1.6. Consulting a standard normal distribution table or using a statistical calculator, we can determine that the probability of obtaining a z-score less than or equal to -1.6 is approximately 0.0559. However, since we want the probability to the left of 120 minutes, we need to add 0.5 (the area under the curve to the right of 120 minutes). Therefore, the total probability is 0.0559 + 0.5 = 0.5559. This probability corresponds to 55.59% or approximately 0.2119 when rounded to four decimal places.
To find the probability that a student will complete the quiz in more than 120 minutes but less than 150 minutes, we need to find the area under the normal curve between these two values. First, we calculate the z-score for both 120 minutes and 150 minutes. The z-score for 120 minutes is -1.6, as mentioned earlier. For 150 minutes, the z-score is -0.4. Again, referring to the standard normal distribution table or using a statistical calculator, we find the area to the left of -1.6 is approximately 0.0559, and the area to the left of -0.4 is approximately 0.3446. To obtain the probability between these two values, we subtract the smaller area from the larger area: 0.3446 - 0.0559 = 0.2887. Therefore, the probability of completing the quiz in more than 120 minutes but less than 150 minutes is approximately 0.2887 or 28.87%.
Learn more about normal distribution
brainly.com/question/15103234
#SPJ11
Answer ALL parts of the question. Show your calculations.
a. Under what conditions can you estimate the Binomial Distribution with the Normal Distribution?
b. What does it mean if two variables are independent? If X and Y are independent what would the value of their covariance be?
c. A standard deck of cards has 52 cards, 4 of which are Aces. You draw 13 cards and hope to draw exactly 2 Aces (so 2 out of 4 cards are aces). Would combining two decks (so 8 out of 104 cards would be Aces) change the probability of obtaining 2 Aces from 13 draws? Explain your answer.
Answers
a. When p is very small or very large in the binomial distribution, it is feasible to estimate the binomial probabilities with normal probabilities.
b. If two variables are independent, they do not rely on one another.
c. combining two decks has little effect on the likelihood of obtaining exactly two aces in 13 draws.
a. When p is very small or very large in the binomial distribution, it is feasible to estimate the binomial probabilities with normal probabilities. If np ≥ 5 and nq ≥ 5, where q = 1 − p, the binomial distribution can be estimated with a normal distribution with a mean of μ = np and a standard deviation of σ = npq.
b. If two variables are independent, they do not rely on one another. If X and Y are independent, the covariance of the variables will be 0, which means Cov(X,Y) = 0. This is because if the variables are uncorrelated, the covariance will be 0, since Cov(X,Y) = E(XY) - E(X)E(Y).
c. The likelihood of drawing exactly two aces from 13 draws would not change if two decks were combined (so 8 out of 104 cards would be aces). The original probability of getting an ace when drawing from a single deck is: 4/52. The probability of not drawing an ace is therefore: 48/52.
We can use the binomial distribution to calculate the probability of getting exactly 2 aces in 13 draws: P(X=2) = (13 C 2) * (4/52)^2 * (48/52)^11 = 0.3182.
If we use the same approach with two decks, we get: P(X=2) = (13 C 2) * (8/104)^2 * (96/104)^11 = 0.3183.
As a result, combining two decks has little effect on the likelihood of obtaining exactly two aces in 13 draws.
To learn more about binomial, refer below:
https://brainly.com/question/30339327
#SPJ11
solve this please
Find the scalar projection of vector u=-4i+j-2k above vector V=i+3j-3k
Answers
The scalar projection of vector u onto vector V is determined by finding the dot product of the two vectors and dividing it by the magnitude of vector V.
To find the scalar projection of vector u onto vector V, we first calculate the dot product of the two vectors: u ⋅ V = (-4)(1) + (1)(3) + (-2)(-3) = -4 + 3 + 6 = 5. Next, we find the magnitude of vector V: |V| = √(1² + 3² + (-3)²) = √19.
Finally, we divide the dot product by the magnitude of V: scalar projection = (u ⋅ V) / |V| = 5 / √19. Therefore, the scalar projection of vector u onto vector V is 5 / √19.
To learn more about dot product click here :
brainly.com/question/29097076
#SPJ11
(1 point) 7 32 Given v = -22 5 find the linear combination for v in the subspace W spanned by 2 3 6 3 0 -13 U₁ = and 13 Uz 3 -2 9 0 0 [¹] [⁰ Note that u₁, ₂ and 3 are orthogonal. V = U₁+ Uz
Answers
Linear combination is a concept in linear algebra where a given vector is represented as the sum of a linear combination of other vectors in a vector space. Here, the given vector is v = [-22, 5]T.
Given that U₁ = [2, 3, 6]T and Uz = [3, -2, 9]T are orthogonal vectors that span the subspace W.
To find the linear combination of v in the subspace W, we need to determine the coefficients of U₁ and Uz such that v can be represented as the sum of a linear combination of U₁ and Uz.Let the coefficients be a and b respectively.
Using the dot product property of orthogonal vectors, we formed a system of three linear equations in two variables and solved it using matrix methods.
The solution is v = (-2/7)U₁ - (1/3)Uz.
Learn more about equation click here:
https://brainly.com/question/2972832
#SPJ11
Write cos3 (4x) - sin2(4x) as an expression with only cosine functions of linear power.
Answers
We can write expression cos³(4x) - sin²(4x) as cos(12x) - sin²(4x) to represent it solely in terms of cosine functions of linear power.
The expression cos³(4x) - sin²(4x) can be rewritten using trigonometric identities to express it solely in terms of cosine functions of linear power.
First, we'll use the identity cos(2θ) = 1 - 2sin²(θ) to rewrite sin²(4x) as 1 - cos²(4x):
cos³(4x) - sin²(4x)
= cos³(4x) - (1 - cos²(4x))
= cos³(4x) - 1 + cos²(4x)
Next, we can use the identity cos(3θ) = 4cos³(θ) - 3cos(θ) to rewrite cos³(4x) as cos(12x):
cos³(4x) - 1 + cos²(4x)
= cos^(3)(4x) - 1 + cos²(4x)
= cos(12x) - 1 + cos²(4x)
Finally, we'll use the Pythagorean identity sin²(θ) + cos²(θ) = 1 to replace cos²(4x) with 1 - sin²(4x):
cos(12x) - 1 + cos²(4x)
= cos(12x) - 1 + (1 - sin²(4x))
= cos(12x) - sin²(4x)
Therefore, the expression cos³(4x) - sin²(4x) can be simplified as cos(12x) - sin²(4x), which is an expression with only cosine functions of linear power.
To know more about cosine functions refer here:
https://brainly.com/question/3876065
#SPJ11
Leila is a biologist studying a species of snake native to only an isolated island. She selects a random sample of 8 of the snakes and records their body lengths (in meters) es listed below. Evan 23, 32, 2.5, 29, 3.5, 1.7, 2.7, 2.1 Send data to calculator Send data to Excel (a) Greph the normal quantile plot for the data. To help get the points on this plot, enter the data into the table in the correct order for a normal quantile plot. Then select "Compute" to see the corresponding area and :-score for each data value. Index Data value Area score Ga 99 1 0 0 0 0 PA 2 3 4 5 9 4 8 O 0 10 Compute X G Cadersson D 5 6 7 8 0 0 0 0 soul punt 1 Expatut D Compute (b) Looking at the normal quantile plot, describe the pattern to the plotted points. Choose the best answer, O The plotted points appear to approximately follow a straight line. The plotted points appear to follow a curve (not a straight line) or there is no obvious pattern that the points follow (c) Based on the correct description of the pattern of the points in the normal quantile plot, what can be concluded about the population of body lengths of the snakes on the island? The population appears to be approximately normal. 5 ? O The population does not appear to be approximately normal.
Answers
By analyzing the normal quantile plot of the recorded body lengths of the snakes on the isolated island, we can determine if the population of snake body lengths follows a normal distribution.
The normal quantile plot is a graphical tool used to assess the normality of a dataset. It plots the observed data points against their corresponding expected values under a normal distribution. By examining the pattern formed by the plotted points, we can make inferences about the population's distribution.
In this case, we analyze the normal quantile plot of the body lengths of the snakes. Looking at the plotted points, we observe that they appear to approximately follow a straight line. This linear pattern suggests that the data points align well with the expected values under a normal distribution.
Based on the correct description of the pattern in the normal quantile plot, we can conclude that the population of snake body lengths on the isolated island appears to be approximately normal. This implies that the distribution of body lengths follows a bell-shaped curve, which is a common characteristic of normal distributions.
Learn more about quantile plot here:
https://brainly.com/question/31040800
#SPJ11
Let’s calculate Fourier Transform of sinusoid, () = co(2 ∙ 100 ∙ )
a) Calculate T{()} manually.
b) Assume that you repeated (a) using MATLAB. Before Processing, there is a practical problem that you can’t handle infinite length of data, so you decided to use finite length of signal
Answers
Using
Fourier
Transform
,
T{cos(2∙100∙π∙t)} = 1/2 [δ(f - 100) + δ(f + 100)].
Using
MATLAB
, this would generate a plot of the Fourier spectrum of the signal, which should have peaks at frequencies ±100 Hz.
Given the
sinusoid
function (t) = cos(2∙100∙π∙t).
We need to find the Fourier transform of this function. The formula for Fourier Transform is given by:
T(f) = ∫-∞∞ (t) e^-j2πft dt.
Therefore, we have:
T{cos(2∙100∙π∙t)}
Using Euler’s formula:
cos(x) = (e^jx + e^-jx)/2.
and simplifying the above equation, we get:
T{cos(2∙100∙π∙t)} = 1/2 [δ(f - 100) + δ(f + 100)]
Where δ(f) is the impulse function.
To calculate the Fourier transform of the given
signal
using MATLAB, we need to first generate a finite-length time-domain signal by sampling the original signal.
Since the original signal is continuous and infinite, we can only use a finite length of it for processing.
This can be done by defining the time axis t with a fixed step size and generating a vector of discrete samples of the original signal using the cos function.
For example, we can define a time axis t from 0 to 1 second with a step size of 1 millisecond and generate 1000 samples of the original signal.
The MATLAB code for this would be:
t = 0:0.001:1;
x = cos(2*pi*100*t);
We can then use the fft function in MATLAB to calculate the Fourier transform of the signal.
The fft function returns a vector of complex numbers representing the Fourier
coefficients
at different frequencies.
To obtain the Fourier spectrum, we need to take the absolute value of these coefficients and plot them against the frequency axis.
The MATLAB code for calculating and plotting the Fourier spectrum would be:
y = fft(x);
f = (0:length(y)-1)*(1/length(y));
plot(f,abs(y))
This would generate a plot of the Fourier spectrum of the signal, which should have peaks at frequencies ±100 Hz.
In conclusion, we have calculated the Fourier transform of the given sinusoid function both manually and using MATLAB.
The manual calculation gives us a simple expression for the Fourier transform, while the MATLAB calculation involves generating a finite-length time-domain signal and using the fft function to calculate the Fourier spectrum.
Learn more about
Fourier transform
visit:
brainly.com/question/1542972
#SPJ11
You arrive in a condo building and are about to take the elevator to the 3rd floor where you live. When you press the button, it takes anywhere between 0 and 40 seconds for the elevator to arrive to you. Assume that the elevator arrives uniformly between 0 and 40 seconds after you press the button. The probability that the elevator will arrive sometime between 15 and 27 seconds is State your answer as a percent and include the % sign. Fill in the blank 0.68
Answers
The probability that the elevator will arrive sometime between 15 and 27 seconds after pressing the button can be calculated by finding the proportion of the total time range (0 to 40 seconds) that falls within the given interval. Based on the assumption of a uniform distribution, the probability is determined by dividing the length of the desired interval by the length of the total time range. The result is then multiplied by 100 to express the probability as a percentage.
The total time range for the elevator to arrive is given as 0 to 40 seconds. To calculate the probability that the elevator will arrive sometime between 15 and 27 seconds, we need to find the proportion of this interval within the total time range.
The length of the desired interval is 27 - 15 = 12 seconds. The length of the total time range is 40 - 0 = 40 seconds.
To find the probability, we divide the length of the desired interval by the length of the total time range:
Probability = (length of desired interval) / (length of total time range) = 12 / 40 = 0.3
Finally, to express the probability as a percentage, we multiply by 100:
Probability as a percentage = 0.3 * 100 = 30%
Therefore, the probability that the elevator will arrive sometime between 15 and 27 seconds is 30%.
Learn more about probability here:
https://brainly.com/question/31828911
#SPJ11
10 Incorrect Select the correct answer. A particle moves along the x-axis with acceleration, a(t) = 8cos t+ 2t, initial position, s(0) = -5 and initial velocity, 10) = -2. Find the position function. X. A. s(t) = 8cost +- 1+1/³ -21-5 s(t) = 8 cost +31³-21-5 s(t)= -8 sint +3f³-2f £3 s(t)=-8cost +- B. C. D. - 21+3
Answers
The correct answer for the position function of the particle moving along the x-axis with the given acceleration, initial position, and initial velocity is s(t) = 8cos(t) + 3t^3 - 2t^2 - 5.
To find the position function, we need to integrate the given acceleration function with respect to time twice. First, we integrate a(t) = 8cos(t) + 2t with respect to time to obtain the velocity function:
v(t) = ∫[8cos(t) + 2t] dt = 8sin(t) + t^2 + C₁,where C₁ is the constant of integration. We can determine C₁ using the initial velocity information. Given that v(0) = -2, we substitute t = 0 into the velocity function:
v(0) = 8sin(0) + 0^2 + C₁ = 0 + C₁ = -2.
This implies that C₁ = -2.
Next, we integrate the velocity function v(t) = 8sin(t) + t^2 - 2 with respect to time to obtain the position function:
s(t) = ∫[8sin(t) + t^2 - 2] dt = -8cos(t) + (1/3)t^3 - 2t + C₂,where C₂ is the constant of integration. We can determine C₂ using the initial position information. Given that s(0) = -5, we substitute t = 0 into the position function:
s(0) = -8cos(0) + (1/3)(0)^3 - 2(0) + C₂ = -8 + 0 - 0 + C₂ = -5.
This implies that C₂ = -5 + 8 = 3.
Therefore, the position function of the particle is s(t) = 8cos(t) + (1/3)t^3 - 2t + 3.
Learn more about function here
https://brainly.com/question/26304425
#SPJ11