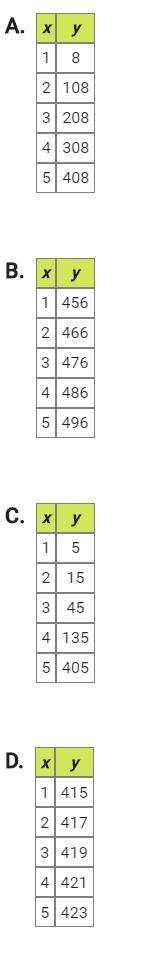
These tables of values represent continuous functions. For which function will the y-values be the greatest for very large values of x?

Answer: D.

Step-by-step explanation:

You want to cube each term in the parentheses. When you take an exponent to an exponent, you just multiply them. You get (27m^-12). In order to get rid of that negative exponent, you should put a one over the m term and make -12, +12:

[tex](\frac{27}{m^12} )(3m^5)[/tex]

Multiply the numerators to get:

[tex]81m^5[/tex]

You now have:

[tex]\frac{81m^5}{m^12}[/tex]

When you divide exponents, you subtract them. 5 - 12 = -7

You have m^-7 which is the same as 1/m^7. Finally, mulitply that by the 81 we left out to get the answer of D.

The expression equivalent to the given expression (3m⁻⁴)³(3m⁵) is 81/m⁷. So, option D is correct.

Exponentiation is a mathematical operation that involves two numbers, the base b, and the exponent or power n, and is pronounced as "b raised to the power of n." It is written as bⁿ and is pronounced as "b raised to the power of n."

When n is a positive integer, bⁿ = b × b × b ×...× b

and b⁻ⁿ = 1/bⁿ

If n = 0, then b⁰ = 1.

Here, (3m⁻⁴)³(3m⁵) = (3³)(m⁻⁴)³(3m⁵) = (27m⁻¹²)(3m⁵) = 81m⁵⁻¹² = 81/m⁷

Therefore, the expression equivalent to the given expression (3m⁻⁴)³(3m⁵) is 81/m⁷. So, option D is correct.

Learn more about exponentiation here -

https://brainly.com/question/14513824

#SPJ2

Answer:

5 hours and 40 minutes would be class

Step-by-step explanation:

We know that the total time is 7 hours, which in minutes would be:

7 * 60 = 420

420 minutes would be class, now, we subtract the other times that are not to be in class and it would be:

420 - 30 - 50 = 340

So we could say that in class it takes 340 minutes, and if we spend hours it would be:

340/60 = 5.67 hours or also 5 hours and 40 minutes would be class.

Answer:

x= -19

Step-by-step explanation:

2x-4x-9=29

-2x=29+9

x=38/-2

= -19

Answer:

[tex]x=2-\sqrt{42}[/tex] and [tex]x=2+\sqrt{42} \\[/tex]

Step-by-step explanation:

Solve using the quadratic formula, which is [tex]x=\frac{-b + \sqrt{b^{2}-4ac }}{2a}[/tex] and [tex]x=\frac{-b-\sqrt{b^{2}-4ac } }{2a}[/tex]

Answer:

[tex]x=1\\x=-1[/tex]

Step-by-step explanation:

[tex]9x^{4} -2x^{2} -7=0\\y=x^{2} \\9y^{2} -2y-7=0\\y=\frac{2\pm\sqrt{(-2)^{2} -4*9(-7)} }{2*9} =\frac{2\pm\sqrt{4+252} }{18} =\frac{2\pm\sqrt{256} }{18}[/tex]

[tex]\sqrt{256} =16[/tex]

[tex]y=\frac{2+16}{18} =\frac{18}{18} =1 \\or \\y=\frac{2-16}{18} =-\frac{14}{18} =-\frac{7}{9}[/tex]

[tex]x^{2} = 1 \\or \\x^{2} =-\frac{7}{9}[/tex]

[tex]x=\pm 1[/tex]

[tex]x^{2} =-\frac{7}{9}[/tex] has no solution since fot all [tex]x[/tex] on the real line, [tex]x^{2} \geq 0[/tex] and [tex]-\frac{7}{9} < 0.[/tex]

Answer:

a) N(2617, 586)

b) 0.3613 = 36.13% probability that the customer consumes less than 2409 calories.

c) 0.4013 = 40.13% of the customers consume over 2764 calories

d) 3981 calories.

Step-by-step explanation:

Problems of normally distributed samples can be solved using the z-score formula.

In a set with mean [tex]\mu[/tex] and standard deviation [tex]\sigma[/tex], the zscore of a measure X is given by:

[tex]Z = \frac{X - \mu}{\sigma}[/tex]

The Z-score measures how many standard deviations the measure is from the mean. After finding the Z-score, we look at the z-score table and find the p-value associated with this z-score. This p-value is the probability that the value of the measure is smaller than X, that is, the percentile of X. Subtracting 1 by the pvalue, we get the probability that the value of the measure is greater than X.

In this question, we have that:

[tex]\mu = 2617, \sigma = 586[/tex]

a. What is the distribution of X?

Here we first place the mean, then the standard deviation.

N(2617, 586)

b. Find the probability that the customer consumes less than 2409 calories.

This is the pvalue of Z when X = 2409. So

[tex]Z = \frac{X - \mu}{\sigma}[/tex]

[tex]Z = \frac{2409 - 2617}{586}[/tex]

[tex]Z = -0.355[/tex]

[tex]Z = -0.355[/tex] has a pvalue of 0.3613

0.3613 = 36.13% probability that the customer consumes less than 2409 calories.

c. What proportion of the customers consume over 2764 calories?

This is 1 subtracted by the pvalue of Z when X = 2764. So

[tex]Z = \frac{X - \mu}{\sigma}[/tex]

[tex]Z = \frac{2764 - 2617}{586}[/tex]

[tex]Z = 0.25[/tex]

[tex]Z = 0.25[/tex] has a pvalue of 0.5987

1 - 0.5987 = 0.4013

0.4013 = 40.13% of the customers consume over 2764 calories

d. The Piggy award will given out to the 1% of customers who consume the most calories. What is the fewest number of calories a person must consume to receive the Piggy award?

Top 1%, so the 100-1 = 99th percentile.

The 99th percentile is the value of X when Z has a pvalue of 0.99. So it is X when Z = 2.327. So

[tex]Z = \frac{X - \mu}{\sigma}[/tex]

[tex]2.327 = \frac{X - 2617}{586}[/tex]

[tex]X - 2617 = 2.327*586[/tex]

[tex]X = 3980.6[/tex]

Rounding to the nearest calorie, 3981 calories.

Answer:

Step-by-step explanation:

To solve this problem, we need to find the number which express the whole park.

Notice that the park is divided in two sections, one occupies 5/8 of the total, and the other occupies 2/7 of the total. So, the sum would be

[tex]\frac{5}{8}+\frac{2}{7}=\frac{35+16}{56} =\frac{51}{56}[/tex]

Now we have the total space there, we need to divide 5/8 by 51/56, so

[tex]\frac{5}{8} \div \frac{51}{56}=\frac{5}{8} \times \frac{56}{51}=\frac{280}{408} \approx 0.69[/tex]

Therefore, the rink occupies 69% of the whole park, approximately, which is equivalent to 280/408.

Step-by-step explanation:

x and x+2 are the numbers

x(x+2)=143

x²+2x-143=0

x²+13x-11x-143=0

x(x+13)- 11(x+13)=0

(x+13). (x-11)=0

x+13=0. x=-13

x-11=0. x=11

Answer:

2044

Step-by-step explanation:

just follow the story

a person uses a

hanger (2)

to pop a

ball  (0)

then flies

two kites (44)

Answer:

1). 2044

Step-by-step explanation:

The story includes: a hanger, a ball, and two kites (in that order)

From the information given, a hanger is 2, a ball is 0, and a kite is 4.

So it would be 2044.

Answer:

a

Step-by-step explanation:

because as absolute value gets smaller the line gets steeper

r

Answer:

y-intercept decreased by 3-1= 2 points

Step-by-step explanation:

y=-9x+ 3 ⇒ y-intercept= 3

y=-9x + 1 ⇒ y-intercept= 1

y-intercept decreased by 3-1= 2 points = the line shifted down by 2 points

Answer:

Hello!

Answer: y-intercept decreased by 2 points because 3-1=2

I hope I was of help.  If not, please let me know!  Thanks!

Step-by-step explanation:

Answer:

Suppose a population of rodents satisfies the differential equation dP 2 kP dt = . Initially there are P (0 2 ) = rodents, and their number is increasing at the rate of 1 dP dt = rodent per month when there are P = 10 rodents.  

How long will it take for this population to grow to a hundred rodents? To a thousand rodents?

Step-by-step explanation:

Use the initial condition when dp/dt = 1, p = 10 to get k;

[tex]\frac{dp}{dt} =kp^2\\\\1=k(10)^2\\\\k=\frac{1}{100}[/tex]

Seperate the differential equation and solve for the constant C.

[tex]\frac{dp}{p^2}=kdt\\\\-\frac{1}{p}=kt+C\\\\\frac{1}{p}=-kt+C\\\\p=-\frac{1}{kt+C} \\\\2=-\frac{1}{0+C}\\\\-\frac{1}{2}=C\\\\p(t)=-\frac{1}{\frac{t}{100}-\frac{1}{2} }\\\\p(t)=-\frac{1}{\frac{2t-100}{200} }\\\\-\frac{200}{2t-100}[/tex]

You have 100 rodents when:

[tex]100=-\frac{200}{2t-100} \\\\2t-100=-\frac{200}{100} \\\\2t=98\\\\t=49\ months[/tex]

You have 1000 rodents when:

[tex]1000=-\frac{200}{2t-100} \\\\2t-100=-\frac{200}{1000} \\\\2t=99.8\\\\t=49.9\ months[/tex]

Answer:

[tex]=13ab[/tex]

Step-by-step explanation:

[tex]5ab+9ab-ab\\\mathrm{Add\:similar\:elements:}\:5ab+9ab-ab=13ab\\=13ab[/tex]

Answer:

multiples of 12

Step-by-step explanation: when looking at the GCF, the answer is 12

Answer:

A)1/18

B)1/6

C)13/18

Step-by-step explanation:

THIS IS THE COMPLETE QUESTION BELOW,

The chess clubs of two schools consist of, respectively, 8 and 9 players. Four members from each club are randomly chosen to participate in a contest between the two schools. The chosen players from one team are then randomly paired with those from the other team, and each pairing plays a game of chess. Suppose that Rebecca and her sister Elise are on the chess clubs at different schools. What is the probability that (a) Rebecca and Elise will be paired? (b) Rebecca and Elise will be chosen to represent their schools but will not play each other? (c) either Rebecca or Elise will be chosen to represent her school?

CHECK THE ATTACHMENT'S FOR STEP BY STEP EXPLANATION

Answer:

360360

Step-by-step explanation:

We have the following information:

Number of ways of choosing 5 car models from 15 different models = 15C5

Number of arranging above 5 models = 5!

Therefore, the total number of displayong 5 models would be:

15C5 * 5!

nCr = n! / (r! * (n-r)!)

we replace:

 15! / (5! * (15-5)!) * 5! = 15! / 10! = 360360

So there are a total of 360 360 ways to display the 5 car models.

Answer:  360,360

Step-by-step explanation:

Answer:

  (0, ∞)

Step-by-step explanation:

An exponential function has a horizontal asymptote at y=0. Its vertical extent is toward infinity.

The range is ...

  0 < f(x) < ∞

Answer:

Yeah btw is this a question?

Answer:

51.56% probability that the mean weight of the sample of cows would differ from the population mean by greater than 11lbs if 49 cows are sampled at random from the herd

Step-by-step explanation:

To solve this question, we need to understand the normal probability distribution and the central limit theorem.

Normal probability distribution

When the distribution is normal, we use the z-score formula.

In a set with mean [tex]\mu[/tex] and standard deviation [tex]\sigma[/tex], the zscore of a measure X is given by:

[tex]Z = \frac{X - \mu}{\sigma}[/tex]

The Z-score measures how many standard deviations the measure is from the mean. After finding the Z-score, we look at the z-score table and find the p-value associated with this z-score. This p-value is the probability that the value of the measure is smaller than X, that is, the percentile of X. Subtracting 1 by the pvalue, we get the probability that the value of the measure is greater than X.

Central Limit Theorem

The Central Limit Theorem estabilishes that, for a normally distributed random variable X, with mean [tex]\mu[/tex] and standard deviation [tex]\sigma[/tex], the sampling distribution of the sample means with size n can be approximated to a normal distribution with mean [tex]\mu[/tex] and standard deviation [tex]s = \frac{\sigma}{\sqrt{n}}[/tex].

For a skewed variable, the Central Limit Theorem can also be applied, as long as n is at least 30.

In this question, we have that:

[tex]\mu = 3181, \sigma = 119, n = 49, s = \frac{119}{\sqrt{49}} = 17[/tex]

What is the probability that the mean weight of the sample of cows would differ from the population mean by greater than 11lbs if 49 cows are sampled at random from the herd

Lower than 3181 - 11 = 3170 lbs or greater than 3181 + 11 = 3192 lbs. Since the normal distribution is symmetric, these probabilities are equal. So i will find one of them, and multiply by 2.

Probability of mean weight lower than 3170 lbs:

This is 1 subtracted by the pvalue of Z when X = 3170. So

[tex]Z = \frac{X - \mu}{\sigma}[/tex]

By the Central Limit Theorem

[tex]Z = \frac{X - \mu}{s}[/tex]

[tex]Z = \frac{3170 - 3181}{17}[/tex]

[tex]Z = -0.65[/tex]

[tex]Z = -0.65[/tex] has a pvalue of 0.2578

2*0.2578 = 0.5156

51.56% probability that the mean weight of the sample of cows would differ from the population mean by greater than 11lbs if 49 cows are sampled at random from the herd

Answer:

The estimated temperature at the point (2.03, 0.96) is 131

Step-by-step explanation:

In this question, we are to estimate the temperature at the given point using the temperature of the unevenly heated plate.

We proceed as follows;

In the question, we identify that the temperature at the point T(2,1) = 130 degrees celcius

Now, let’s look at how the temperature changes. There is a positive change of 16 units when we move across the x-axis and a negative decrease when we move up the y-axis to a tune of 16 units(negative)

Now, how does the problem wants us to move using the notation of change?

Look at the point (2.03, 0.96), since movement across the x-axis is positive, the motion here in terms of x i.e Δx is 0.03 while the corresponding motion in terms of y(albeit negative) is Δy = -0.04

Mathematically, the change in temperature is proportional to the distance traveled. What this means is that we need to multiply the changes in direction by the corresponding temperature. This is shown below;

ΔTx =Δx*Tx(2,1) => ΔTx = (0.03)*(16) = 0.48

ΔTy = Δy*Ty(2,1) => ΔTy = (-0.04)*(-13) = 0.52

We can now combine the equations above to form a single one as follows; which is an approximation;

ΔT = Δx*Tx(2,1) + Δy*Ty(2,1) => ΔT = (0.03)*(16) + (-0.04)*(-13) = 1

To arrive at the final answer, we add the change in temperature to the staring temperature which is ;

T(2.03,0.96) = T(2,1) + ΔT = 130 + 1= 131

Answer:

90% confidence interval for the true proportion of men who applied to the nursing   program.​

(0.09674 ,0.14326)

Step-by-step explanation:

Explanation:-

Given sample size 'n' = 500

sample proportion

                [tex]p = \frac{x}{n} = \frac{60}{500} = 0.12[/tex]

Level of significance ∝= 0.90 or 0.10

90% confidence interval for the true proportion of men who applied to the nursing   program.​

[tex](p - Z_{\frac{0.10}{2} } \sqrt{\frac{p(1-p)}{n} } , p + Z_{\frac{0.10}{2} } \sqrt{\frac{p(1-p)}{n} })[/tex]

[tex](p - Z_{0.05 } \sqrt{\frac{p(1-p)}{n} } , p + Z_{0.05 } \sqrt{\frac{p(1-p)}{n} })[/tex]

[tex](0.12 - 1.645 \sqrt{\frac{0.12(1-0.12)}{500} } , 0.12 + 1.645 \sqrt{\frac{0.12(1-0.12)}{500} })[/tex]

On calculation , we get

( 0.12 - 0.02326 , 0.12 + 0.02326)

(0.09674 ,0.14326)

Final answer:-

90% confidence interval for the true proportion of men who applied to the nursing   program.​

(0.09674 ,0.14326)

Answer:

[tex]x=5/48[/tex]

Step-by-step explanation:

[tex]2/5 + 1/x =10[/tex]

[tex]1/x=10-2/5[/tex]

[tex]1/x=48/5[/tex]

[tex]48x=5[/tex]

[tex]x=5/48[/tex]

Answer:

[tex]x = \frac{5}{48} [/tex]

Step-by-step explanation:

[tex]\frac{2}{5} + \frac{1}{x} = 10 \\ \frac{1}{x} = 10 - \frac{2}{5} \\ \frac{1}{x} = \frac{50 - 2}{5} \\ \frac{1}{ x } = \frac{48}{5} \\

use \: \: \: \: cross \: \: \: multiply

\\ 5 = 48x \\ \frac{5}{48} = \frac{48x}{48} \\ x = \frac{5}{48} \\ [/tex]

hope this helps

brainliest appreciated

good luck! have a nice day!

Answer:

The answer would 177.06 centimeters

Answer:

b) False

Explanation:

It is not needed to keep 50/50 split.

Answer:

$9400

Step-by-step explanation:

1.5x4=6%

100-6=94

0.94x10000=9400

Answer:

4. 27

Step-by-step explanation:

11-10=1 which is <=16

15-10=5 which is <=16

26-10=16 which is <=16

27-10=17 which isn't <=16

Therefore 27 doesn't satisfy the inequality

Answer:

4.   27

Step-by-step explanation:

w - 10 ≤ 16

w≤16 + 10

w ≤ 26

11 ≤ 26

15≤26

26≤26

26≤ 27 False

Step-by-step explanation:

p = x / n

p = 550 / 1083

p = 0.5078

Answer:

the Swiss Chalet had higher occupancy than its competitive set in 2019

Step-by-step explanation:

Answer: 5^5

Step-by-step explanation:

Since 5x5x5x5x5 = 3,125

Answer:

  see below

Step-by-step explanation:

The first subtraction has a zero result (blue) from the thousands digit, so we know the dividend has 4 in that place. The 5 in the 1s place of the dividend is brought down to fill the space on the bottom line. 6 goes into that number 0 times, so the final quotient digit is 0.

  4,925 = 6×820 +5

or

  4,925 ÷ 6 = 820 r5